
A chaotic electromagnetic whirl contains information about charge and mass, even before matter itself has developed. The latter, despite being made up of individual particles, emerges from the aforementioned background radiation and forms a whole with it, which follows precise time-evolution laws.


1. Indipendentemente dal fatto che la materia esista o meno, un oceano di onde elettromagnetiche riempie l’intero universo senza lasciare lacune. Un ribollio di campi elettrici e magnetici si evolve in complesse strutture che si compongono e, pochi istanti dopo, si ridistruggono, come tra i flutti di un mare in tempesta. (continua)

2. Le equazioni differenziali che descrivono il comportamento del sottofondo si ottengono accoppiando opportunamente quelle dell’elettrodinamica con quelle della fluidodinamica. Tale è la prassi in molte circostanze, anche se il modello qui proposto è leggermente differente da quelli classici, per motivi che non stiamo a dettagliare. (continua)
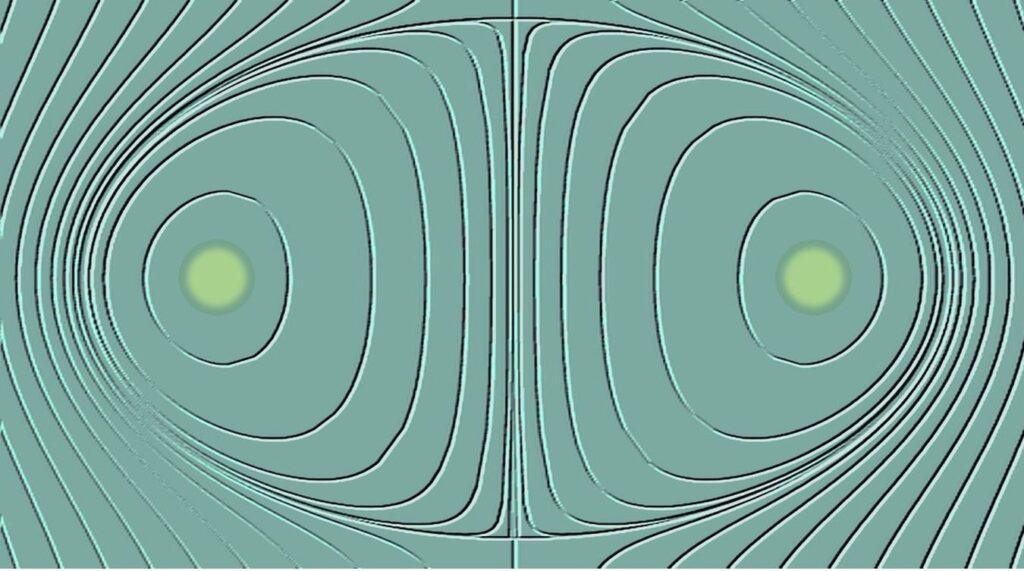
3. E’ possibile costruire soluzioni del modello elettrodinamico che hanno una struttura ad anello. Tali soluzioni sono di fatto presenti in simili modelli che governano la fisica dei plasmi o in magnetoidrodinamica. Tarando opportunamente i parametri a disposizione, si possono ottenere anelli delle dimensioni dei nuclei atomici, i quali portano carica unitaria negativa e massa pari a quella dell’elettrone. (continua)

4. Mentre la costruzione dell’elettrone, a partire dalle equazioni modello, può essere ritenuta abbastanza completa, per quanto riguarda il protone l’esercizio è assai più complesso, perchè di fatto è molto più complessa la struttura di tale particella. Si possono azzardare alcune ipotesi in maniera da rispettare il più possibile le osservazioni sperimentali. (continua)
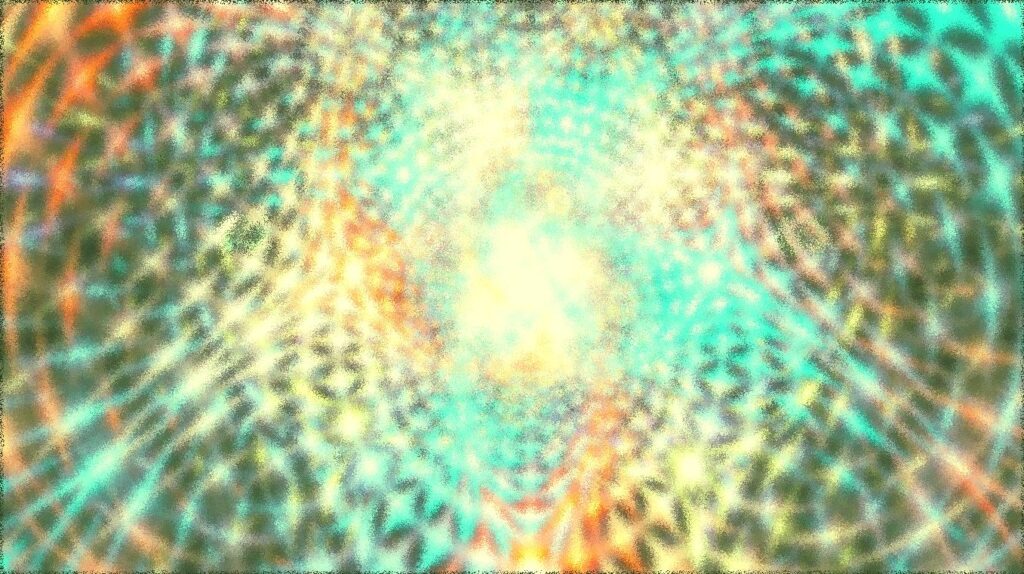
5. Uno dei punti salienti della nostra trattazione è che il sottofondo possa far emergere elementi di vera materia (o anti materia). Detti oggetti sono anch’essi di natura elettromagnetica. L’ipotesi successiva è di ritenere che tali configurazioni possano significativamente incidere sull’evoluzione dei fenomeni elettromagnetici nel loro intorno. (continua)

6. Abbiamo a questo punto tutti gli elementi per affrontare alcuni aspetti più cruciali riguardanti la materia, la quale si presenta come una combinazione di particelle cariche e munite di massa, immerse in un mare agitato di quella che potremmo chiamare pseudo-materia. Quest’ultima non funge solo da sottofondo, ma contribuisce in modo unico nel caratterizzare la struttura delle molecole. (continua)
Quello esposto in queste pagine non è solamente un saggio (più o meno circostanziato) su come possa essere strutturata la matera inanimata al di là dell’interpretazione quantistica (prevalentemente meccanicistica). L’indissolubilità fra il mondo delle particelle e l’ambiente elettromagnetico che le circonda, ci suggeriscono che gli elementi dell’universo siano tutti collegati, in un miscuglio di quantità “solide” inframmezzate da ghirigori elettromagnetici in instancabile movimento. Evidentemente, questa visione ha ripercussioni sulla vita biologica, a partire dalla sua costituzione, nonchè sull’azione che detta organizzazione ha sulle attività umane. Rinviamo per un approfondimento di queste tematiche all’articolo QUA reperibile.
What exposed in these pages is not only a (more or less detailed) essay on how inanimate matter can be structured beyond the quantum interpretation (mainly mechanistic). The indissolubility between the world of particles and the electromagnetic environment that surrounds them, suggests that the elements of the universe are all connected in a mixture of “solid” quantities interspersed with electromagnetic doodles in tireless movement. Evidently, this vision has repercussions on biological life, starting from its constitution, as well as on the action that such an organization has on human activities. For further information on these issues, we refer to the article available HERE.
Draft completed in July 2023. Last update: April 2024.
